Algebra tiles are the coolest little things. They are a wonderful hands-on tool that can be used to visually represent so many topics in algebra, from solving equations to multiplying binomials to factoring quadratic trinomials.
New to algebra tiles? Watch my algebra tiles tutorial video here.

In this post, I want to focus on that last topic -- using algebra tiles to factor quadratic trinomials. Algebra tiles are a perfect way to introduce and practice this concept. They take a lot of the guesswork out of factoring, especially for trinomials that are not easily factored with other methods.
Below are 4 examples of how to use algebra tiles to factor, starting with a trinomial where A=1 (and the B and C values are both positive), all the way to a trinomial with A>1 (and negative B and/or C values).
Here is a look at the tiles in this post:
In my set of algebra tiles, the same-size tiles are double-sided with + on one side and - on the other. You can get a similar effect by printing this free printable set of algebra tiles on astrobrights paper (or glue 2 different colored pieces of paper together back-to-back before cutting).
As much as I love cut-laminate-cut, Teacher Ms. Baker has tested it out and laminate-cut works well for reinforcing paper algebra tiles.
Let's jump into the examples!
Factoring this trinomial is a nice place to start because the A value is 1 and both the B and C values are positive. We'll be able to get a good feel for how the algebra tiles work with this example.
Needed:
1 x2 tile
5 rectangular x tiles
6 + tiles
Here we have all of the tiles we will need to factor this trinomial. Now it's just a matter of putting the puzzle together.
First, I tried (x + 4)(x + 1). This used all of the rectangular x tiles but not all of the + tiles. In order for the trinomial to be factored correctly, all tiles have to fit together to make a perfect rectangle.
Next I tried (x + 2)(x + 3), which allowed all 6 of the + tiles to fit.
x2 + 5x + 6 factors to (x + 2)(x + 3).
Algebra tiles are great for factoring quadratic trinomials where the A value is not 1. I have always liked the AC method for factoring these trinomials, but even I will admit that factoring with algebra tiles in this instance is all around way better.
Needed:
2 x2 tiles
3 rectangular x tiles
1 + tile
Here we have all the tiles we need to factor this trinomial. With trinomials where the A, B and C values are all positive, we start and finish with the same number of tiles. (In a minute when we factor a trinomial where B and/or C are negative, the approach will be slightly different.)
Arranging the algebra tiles into (x + 1)(2x + 1) allowed all of the tiles to fit together into a nice, neat rectangle.
2x2 + 3x + 1 factors to (x + 1)(2x + 1).
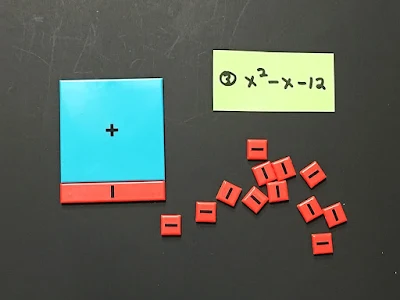
When we factor quadratic trinomials involving negatives, we may not start and end with the same number of tiles. We may have to add in some zero pairs as we go. In the case of x2 - x - 12, our B value is -1.
Needed (to start):
1 x2 tile
1 rectangular -x tile
12 - tiles
Since there was no way to make a rectangle that fit those 12 - tiles, we needed to add in some additional zero pairs (1 of each rectangular + and - x tile).
We can do this because:
-1 + 0 = -1 or
-2 + 1 = -1 or
-3 + 2 = -1 .... and so on...
By adding in equal amounts of rectangular + and - x tiles, we are not changing the trinomial's B value. It's still -1.
Here we have 3 rectangular -x tiles and 2 rectangular x tiles. Still not enough to fit those 12 - tiles, though the B value is still being represented as -1.
We got it! We needed 4 rectangular -x tiles and 3 rectangular x tiles to make all 12 - tiles fit. -4 + 3 - =1 so we did not change the trinomial's B value.
x2 - x - 12 factors to (x - 4)(x + 3).
Right away it's obvious that we do not have enough pieces to make a nice, even rectangle. So we will need to start adding zero pairs to keep B at +1. Here I added a rectangular +x and a rectangular -x. This wasn't quite enough to fill it in.
I added one more zero pair of x tiles and our rectangle is complete!
2x2 + x - 3 factors to (x - 1)(2x + 3).
I had gotten this set of algebra tiles secondhand online. If you don't have a set, you can print this free set of printable algebra tiles.
There are 2 versions of the algebra tiles in the pdf. The first page mirrors the dimensions of my plastic set. The algebra tiles on the second page are a little larger for kids who may have trouble with the smaller size.
Additional resources:
If you'd like to learn more about ways to use algebra tiles, I have put together an algebra tiles tutorial video that covers ways to use algebra tiles in middle school math.
Related posts:
Solving Equations Using Algebra Tiles
Cereal Factoring
Fun with Quadratics

In this post, I want to focus on that last topic -- using algebra tiles to factor quadratic trinomials. Algebra tiles are a perfect way to introduce and practice this concept. They take a lot of the guesswork out of factoring, especially for trinomials that are not easily factored with other methods.
Below are 4 examples of how to use algebra tiles to factor, starting with a trinomial where A=1 (and the B and C values are both positive), all the way to a trinomial with A>1 (and negative B and/or C values).
Here is a look at the tiles in this post:
In my set of algebra tiles, the same-size tiles are double-sided with + on one side and - on the other. You can get a similar effect by printing this free printable set of algebra tiles on astrobrights paper (or glue 2 different colored pieces of paper together back-to-back before cutting).
As much as I love cut-laminate-cut, Teacher Ms. Baker has tested it out and laminate-cut works well for reinforcing paper algebra tiles.
Let's jump into the examples!
Example 1:
Factor x2 + 5x + 6
Factoring this trinomial is a nice place to start because the A value is 1 and both the B and C values are positive. We'll be able to get a good feel for how the algebra tiles work with this example.
Needed:
1 x2 tile
5 rectangular x tiles
6 + tiles
Here we have all of the tiles we will need to factor this trinomial. Now it's just a matter of putting the puzzle together.
First, I tried (x + 4)(x + 1). This used all of the rectangular x tiles but not all of the + tiles. In order for the trinomial to be factored correctly, all tiles have to fit together to make a perfect rectangle.
Next I tried (x + 2)(x + 3), which allowed all 6 of the + tiles to fit.
x2 + 5x + 6 factors to (x + 2)(x + 3).
Example 2:
Factor 2x2 + 3x + 1
Algebra tiles are great for factoring quadratic trinomials where the A value is not 1. I have always liked the AC method for factoring these trinomials, but even I will admit that factoring with algebra tiles in this instance is all around way better.
Needed:
2 x2 tiles
3 rectangular x tiles
1 + tile
Here we have all the tiles we need to factor this trinomial. With trinomials where the A, B and C values are all positive, we start and finish with the same number of tiles. (In a minute when we factor a trinomial where B and/or C are negative, the approach will be slightly different.)
Arranging the algebra tiles into (x + 1)(2x + 1) allowed all of the tiles to fit together into a nice, neat rectangle.
2x2 + 3x + 1 factors to (x + 1)(2x + 1).
Example 3:
Factor x2 - x - 12
When we factor quadratic trinomials involving negatives, we may not start and end with the same number of tiles. We may have to add in some zero pairs as we go. In the case of x2 - x - 12, our B value is -1.
Needed (to start):
1 x2 tile
1 rectangular -x tile
12 - tiles
Since there was no way to make a rectangle that fit those 12 - tiles, we needed to add in some additional zero pairs (1 of each rectangular + and - x tile).
We can do this because:
-1 + 0 = -1 or
-2 + 1 = -1 or
-3 + 2 = -1 .... and so on...
By adding in equal amounts of rectangular + and - x tiles, we are not changing the trinomial's B value. It's still -1.
Here we have 3 rectangular -x tiles and 2 rectangular x tiles. Still not enough to fit those 12 - tiles, though the B value is still being represented as -1.
We got it! We needed 4 rectangular -x tiles and 3 rectangular x tiles to make all 12 - tiles fit. -4 + 3 - =1 so we did not change the trinomial's B value.
x2 - x - 12 factors to (x - 4)(x + 3).
Example 4:
Factor 2x2 + x - 3
Using Algebra tiles is so helpful when factoring quadratic trinomials where the A value is greater than 1 and B and/or C are negative. The tiles make the process so much more intuitive!
Needed (to start):
2 x2 tiles
1 rectangular x tile
3 - tiles
Right away it's obvious that we do not have enough pieces to make a nice, even rectangle. So we will need to start adding zero pairs to keep B at +1. Here I added a rectangular +x and a rectangular -x. This wasn't quite enough to fill it in.
I added one more zero pair of x tiles and our rectangle is complete!
2x2 + x - 3 factors to (x - 1)(2x + 3).
I had gotten this set of algebra tiles secondhand online. If you don't have a set, you can print this free set of printable algebra tiles.
There are 2 versions of the algebra tiles in the pdf. The first page mirrors the dimensions of my plastic set. The algebra tiles on the second page are a little larger for kids who may have trouble with the smaller size.
Additional resources:
If you'd like to learn more about ways to use algebra tiles, I have put together an algebra tiles tutorial video that covers ways to use algebra tiles in middle school math.
Using Algebra Tiles in Middle School Math:
 |
| watch video |
Solving Equations Using Algebra Tiles
Cereal Factoring
Fun with Quadratics
















Iloveit
ReplyDeleteThanks so much! This really made me understand quadratic equations
ReplyDelete